New Support for Alternative Quantum View

Olena Shmahalo/Quanta Magazine
Introduction
Of the many counterintuitive features of quantum mechanics, perhaps the most challenging to our notions of common sense is that particles do not have locations until they are observed. This is exactly what the standard view of quantum mechanics, often called the Copenhagen interpretation, asks us to believe. Instead of the clear-cut positions and movements of Newtonian physics, we have a cloud of probabilities described by a mathematical structure known as a wave function. The wave function, meanwhile, evolves over time, its evolution governed by precise rules codified in something called the Schrödinger equation. The mathematics are clear enough; the actual whereabouts of particles, less so. Until a particle is observed, an act that causes the wave function to “collapse,” we can say nothing about its location. Albert Einstein, among others, objected to this idea. As his biographer Abraham Pais wrote: “We often discussed his notions on objective reality. I recall that during one walk Einstein suddenly stopped, turned to me and asked whether I really believed that the moon exists only when I look at it.”
But there’s another view — one that’s been around for almost a century — in which particles really do have precise positions at all times. This alternative view, known as pilot-wave theory or Bohmian mechanics, never became as popular as the Copenhagen view, in part because Bohmian mechanics implies that the world must be strange in other ways. In particular, a 1992 study claimed to crystalize certain bizarre consequences of Bohmian mechanics and in doing so deal it a fatal conceptual blow. The authors of that paper concluded that a particle following the laws of Bohmian mechanics would end up taking a trajectory that was so unphysical — even by the warped standards of quantum theory — that they described it as “surreal.”
Nearly a quarter-century later, a group of scientists has carried out an experiment in a Toronto laboratory that aims to test this idea. And if their results, first reported earlier this year, hold up to scrutiny, the Bohmian view of quantum mechanics — less fuzzy but in some ways more strange than the traditional view — may be poised for a comeback.
Saving Particle Positions
Bohmian mechanics was worked out by Louis de Broglie in 1927 and again, independently, by David Bohm in 1952, who developed it further until his death in 1992. (It’s also sometimes called the de Broglie–Bohm theory.) As with the Copenhagen view, there’s a wave function governed by the Schrödinger equation. In addition, every particle has an actual, definite location, even when it’s not being observed. Changes in the positions of the particles are given by another equation, known as the “pilot wave” equation (or “guiding equation”). The theory is fully deterministic; if you know the initial state of a system, and you’ve got the wave function, you can calculate where each particle will end up.
That may sound like a throwback to classical mechanics, but there’s a crucial difference. Classical mechanics is purely “local” — stuff can affect other stuff only if it is adjacent to it (or via the influence of some kind of field, like an electric field, which can send impulses no faster than the speed of light). Quantum mechanics, in contrast, is inherently nonlocal. The best-known example of a nonlocal effect — one that Einstein himself considered, back in the 1930s — is when a pair of particles are connected in such a way that a measurement of one particle appears to affect the state of another, distant particle. The idea was ridiculed by Einstein as “spooky action at a distance.” But hundreds of experiments, beginning in the 1980s, have confirmed that this spooky action is a very real characteristic of our universe.
In the Bohmian view, nonlocality is even more conspicuous. The trajectory of any one particle depends on what all the other particles described by the same wave function are doing. And, critically, the wave function has no geographic limits; it might, in principle, span the entire universe. Which means that the universe is weirdly interdependent, even across vast stretches of space. The wave function “combines — or binds — distant particles into a single irreducible reality,” as Sheldon Goldstein, a mathematician and physicist at Rutgers University, has written.
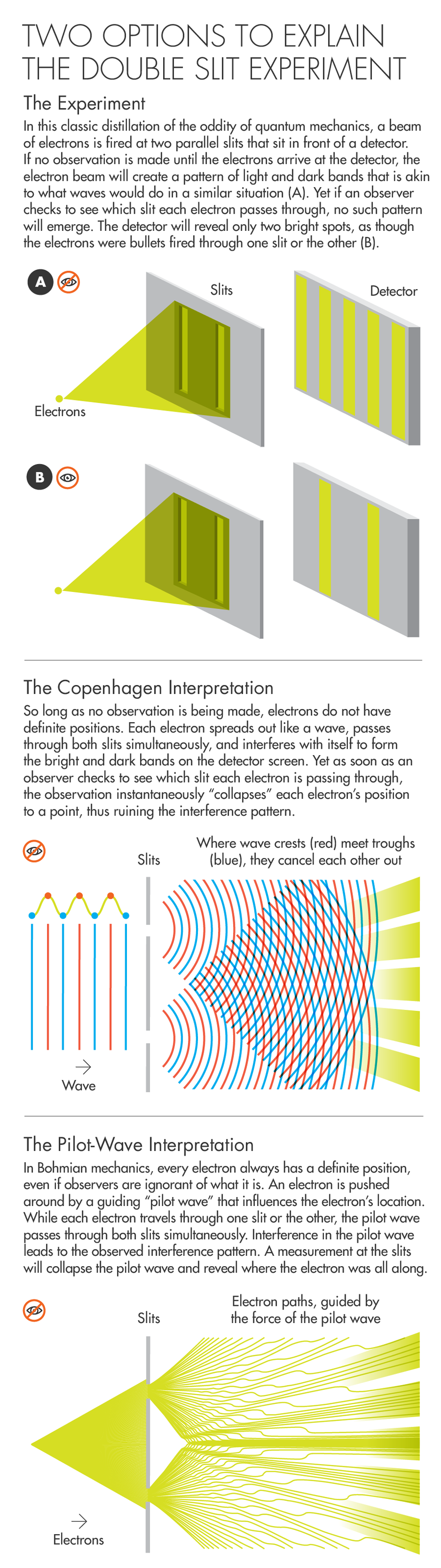
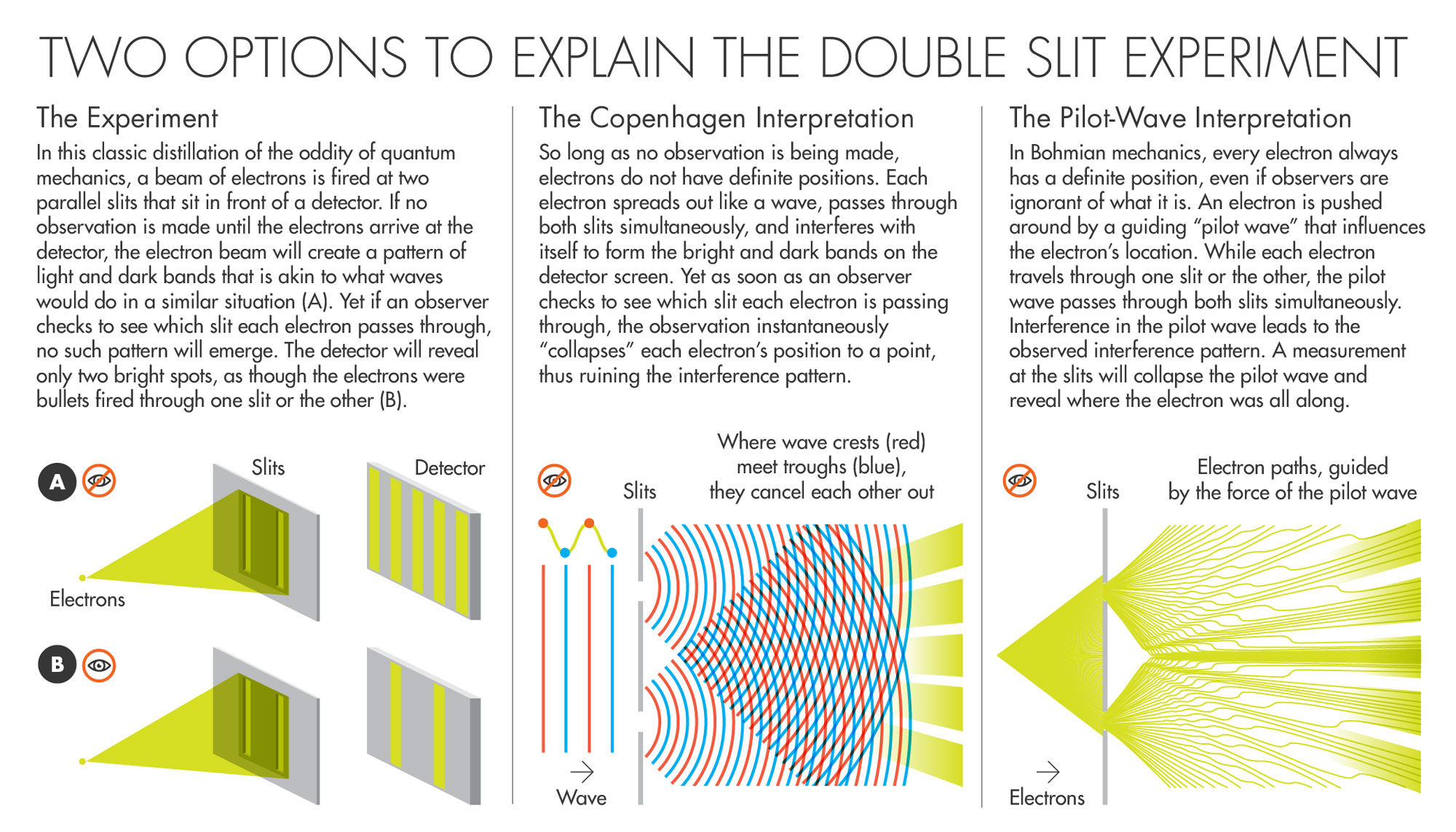
The differences between Bohm and Copenhagen become clear when we look at the classic “double slit” experiment, in which particles (let’s say electrons) pass through a pair of narrow slits, eventually reaching a screen where each particle can be recorded. When the experiment is carried out, the electrons behave like waves, creating on the screen a particular pattern called an “interference pattern.” Remarkably, this pattern gradually emerges even if the electrons are sent one at a time, suggesting that each electron passes through both slits simultaneously.
Those who embrace the Copenhagen view have come to live with this state of affairs — after all, it’s meaningless to speak of a particle’s position until we measure it. Some physicists are drawn instead to the Many Worlds interpretation of quantum mechanics, in which observers in some universes see the electron go through the left slit, while those in other universes see it go through the right slit — which is fine, if you’re comfortable with an infinite array of unseen universes.
By comparison, the Bohmian view sounds rather tame: The electrons act like actual particles, their velocities at any moment fully determined by the pilot wave, which in turn depends on the wave function. In this view, each electron is like a surfer: It occupies a particular place at every specific moment in time, yet its motion is dictated by the motion of a spread-out wave. Although each electron takes a fully determined path through just one slit, the pilot wave passes through both slits. The end result exactly matches the pattern one sees in standard quantum mechanics.
Lucy Reading-Ikkanda for Quanta Magazine
For some theorists, the Bohmian interpretation holds an irresistible appeal. “All you have to do to make sense of quantum mechanics is to say to yourself: When we talk about particles, we really mean particles. Then all the problems go away,” said Goldstein. “Things have positions. They are somewhere. If you take that idea seriously, you’re led almost immediately to Bohm. It’s a far simpler version of quantum mechanics than what you find in the textbooks.” Howard Wiseman, a physicist at Griffith University in Brisbane, Australia, said that the Bohmian view “gives you a pretty straightforward account of how the world is…. You don’t have to tie yourself into any sort of philosophical knots to say how things really are.”
But not everyone feels that way, and over the years the Bohm view has struggled to gain acceptance, trailing behind Copenhagen and, these days, behind Many Worlds as well. A significant blow came with the paper known as “ESSW,” an acronym built from the names of its four authors. The ESSW paper claimed that particles can’t follow simple Bohmian trajectories as they traverse the double-slit experiment. Suppose that someone placed a detector next to each slit, argued ESSW, recording which particle passed through which slit. ESSW showed that a photon could pass through the left slit and yet, in the Bohmian view, still end up being recorded as having passed through the right slit. This seemed impossible; the photons were deemed to follow “surreal” trajectories, as the ESSW paper put it.
The ESSW argument “was a striking philosophical objection” to the Bohmian view, said Aephraim Steinberg, a physicist at the University of Toronto. “It damaged my love for Bohmian mechanics.”
But Steinberg has found a way to rekindle that love. In a paper published in Science Advances, Steinberg and his colleagues — the team includes Wiseman, in Australia, as well as five other Canadian researchers — describe what happened when they actually performed the ESSW experiment. They found that the photon trajectories aren’t surrealistic after all — or, more precisely, that the paths may seem surrealistic, but only if one fails to take into account the nonlocality inherent in Bohm’s theory.
The experiment that Steinberg and his team conducted was analogous to the standard two-slit experiment. They used photons rather than electrons, and instead of sending those photons through a pair of slits, they passed through a beam splitter, a device that directs a photon along one of two paths, depending on the photon’s polarization. The photons eventually reach a single-photon camera (equivalent to the screen in the traditional experiment) that records their final position. The question “Which of two slits did the particle pass through?” becomes “Which of two paths did the photon take?”
Importantly, the researchers used pairs of entangled photons rather than individual photons. As a result, they could interrogate one photon to gain information about the other. When the first photon passes through the beam splitter, the second photon “knows” which path the first one took. The team could then use information from the second photon to track the first photon’s path. Each indirect measurement yields only an approximate value, but the scientists could average large numbers of measurements to reconstruct the trajectory of the first photon.
The team found that the photon paths do indeed appear to be surreal, just as ESSW predicted: A photon would sometimes strike one side of the screen, even though the polarization of the entangled partner said that the photon took the other route.
But can the information from the second photon be trusted? Crucially, Steinberg and his colleagues found that the answer to the question “Which path did the first photon take?” depends on when it is asked.
At first — in the moments immediately after the first photon passes through the beam splitter — the second photon is very strongly correlated with the first photon’s path. “As one particle goes through the slit, the probe [the second photon] has a perfectly accurate memory of which slit it went through,” Steinberg explained.
But the farther the first photon travels, the less reliable the second photon’s report becomes. The reason is nonlocality. Because the two photons are entangled, the path that the first photon takes will affect the polarization of the second photon. By the time the first photon reaches the screen, the second photon’s polarization is equally likely to be oriented one way as the other — thus giving it “no opinion,” so to speak, as to whether the first photon took the first route or the second (the equivalent of knowing which of the two slits it went through).
The problem isn’t that Bohm trajectories are surreal, said Steinberg. The problem is that the second photon says that Bohm trajectories are surreal — and, thanks to nonlocality, its report is not to be trusted. “There’s no real contradiction in there,” said Steinberg. “You just have to always bear in mind the nonlocality, or you miss something very important.”
Faster Than Light
Some physicists, unperturbed by ESSW, have embraced the Bohmian view all along and aren’t particularly surprised by what Steinberg and his team found. There have been many attacks on the Bohmian view over the years, and “they all fizzled out because they had misunderstood what the Bohm approach was actually claiming,” said Basil Hiley, a physicist at Birkbeck, University of London (formerly Birkbeck College), who collaborated with Bohm on his last book, The Undivided Universe. Owen Maroney, a physicist at the University of Oxford who was a student of Hiley’s, described ESSW as “a terrible argument” that “did not present a novel challenge to de Broglie–Bohm.” Not surprisingly, Maroney is excited by Steinberg’s experimental results, which seem to support the view he’s held all along. “It’s a very interesting experiment,” he said. “It gives a motivation for taking de Broglie–Bohm seriously.”
On the other side of the Bohmian divide, Berthold-Georg Englert, one of the authors of ESSW (along with Marlan Scully, George Süssman and Herbert Walther), still describes their paper as a “fatal blow” to the Bohmian view. According to Englert, now at the National University of Singapore, the Bohm trajectories exist as mathematical objects but “lack physical meaning.”
On a historical note, Einstein lived just long enough to hear about Bohm’s revival of de Broglie’s proposal — and he wasn’t impressed, dismissing it as too simplistic to be correct. In a letter to physicist Max Born, in the spring of 1952, Einstein weighed in on Bohm’s work:
Have you noticed that Bohm believes (as de Broglie did, by the way, 25 years ago) that he is able to interpret the quantum theory in deterministic terms? That way seems too cheap to me. But you, of course, can judge this better than I.
But even for those who embrace the Bohmian view, with its clearly defined particles moving along precise paths, questions remain. Topping the list is an apparent tension with special relativity, which prohibits faster-than-light communication. Of course, as physicists have long noted, nonlocality of the sort associated with quantum entanglement does not allow for faster-than-light signaling (thus incurring no risk of the grandfather paradox or other violations of causality). Even so, many physicists feel that more clarification is needed, especially given the prominent role of nonlocality in the Bohmian view. The apparent dependence of what happens here on what may be happening there cries out for an explanation.
“The universe seems to like talking to itself faster than the speed of light,” said Steinberg. “I could understand a universe where nothing can go faster than light, but a universe where the internal workings operate faster than light, and yet we’re forbidden from ever making use of that at the macroscopic level — it’s very hard to understand.”
This article was reprinted on Wired.com.