Solution: ‘Bongard Problems and Scientific Discovery’
This month’s puzzle featured inductive visual Bongard problems that mimic the process of scientific reasoning. Besides the traditional geometric puzzles, we also added some inductive number and word problems. As in scientific problems found in nature, Bongard-type problems give us exemplars that follow a particular unknown rule or pattern and others that do not follow it, or follow a different or complementary rule. We must discover the correct rule, which can lead us through a frustrating blind search before possibly rewarding us with an “aha!” moment when we find a simple, elegant rule that fits. In science, of course, we can create our own experimental exemplars and query nature with them to see what group they belong to. In Bongard problems, we have no such recourse, unless we have a continuous dialogue with the designer.
Here are the answers to the problems:
Problem 1
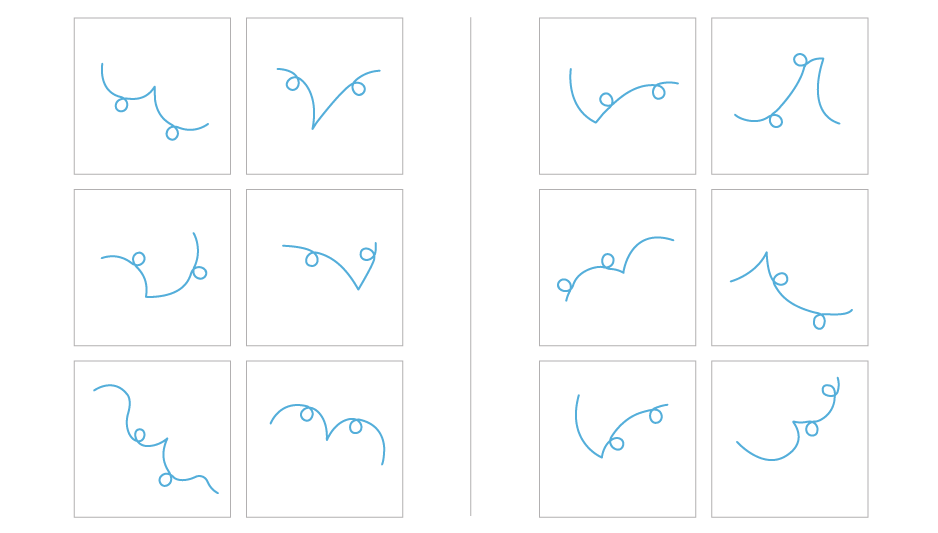
Courtesy of Harry Foundalis. Bongard Problem 44 (Designer: M.M. Bongard)
All the drawings have a kink and two loops. The figures on the left have a kink between the two loops; those on the right have both loops on one side of the kink.
Problem 2
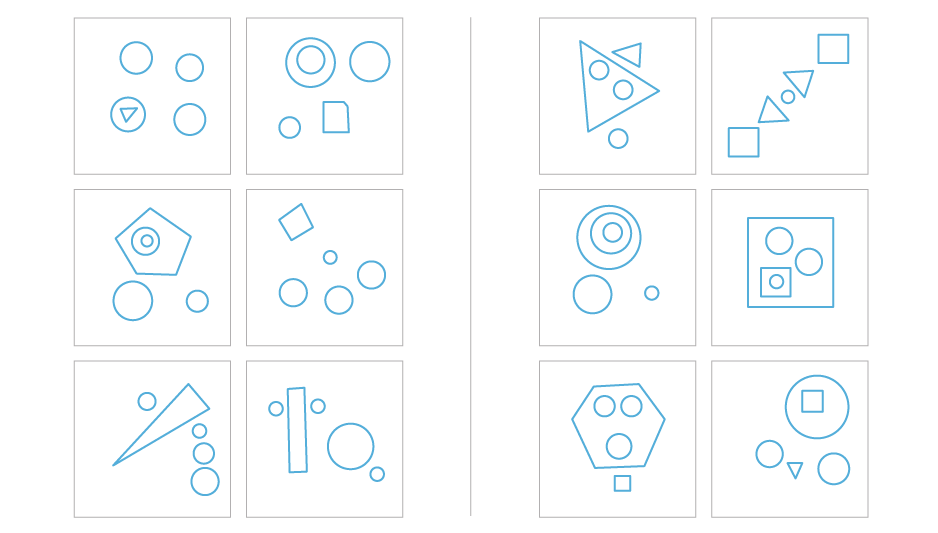
Courtesy of Harry Foundalis. Bongard Problem 110 (Designer: Douglas Hofstadter)
All the figures contain five geometric shapes. In the ones on the left, four of these shapes are circles. The ones on the right do not have four circles.
Problem 3
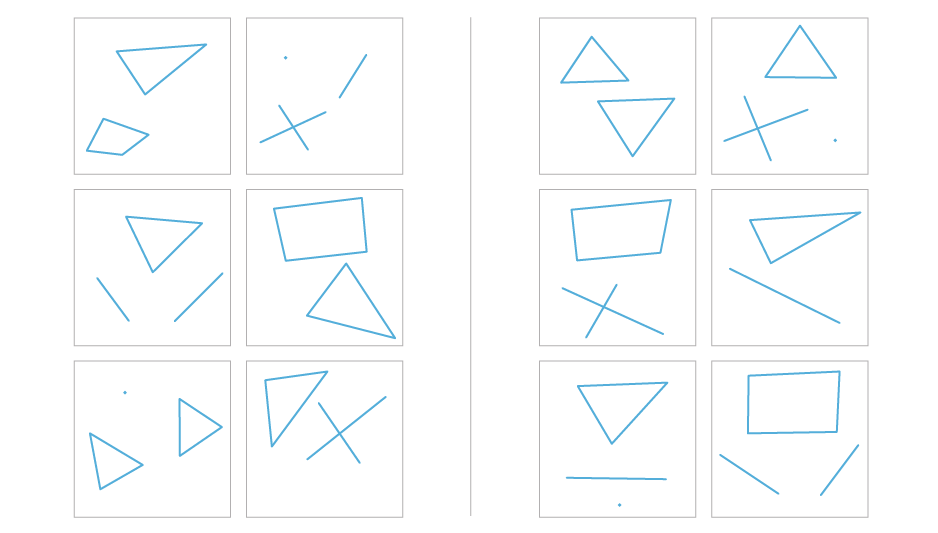
Courtesy of Harry Foundalis. Bongard Problem 230 (Designer: Joseph A.L. Insana)
The figures here can be generated by a bunch of scattered points, all of which may or may not be connected by straight lines. In the left panel, all the figures were generated from exactly seven points, or as Douglas Felix put it in mathematical terms, they are graphs of order 7. The figures on the right were generated using a different number of points.
Problem 4
Follow the rule: 65 12 47 38 96 29
Don’t follow the rule: 53 26 93 19 68 37
Both groups consist of two digit numbers. In the top group, the two digits have opposite parity (one is odd, the other is even). In the bottom group, both digits have the same parity. This rule also implies that the sum or difference of the two digits is odd in the case of the first group, and even in the case of the second.
Problem 5
Follow the rule: 50 58 21 74 91 78
Don’t follow the rule: 11 26 35 57 48 88
The rule here required writing the numbers in Roman notation. The first group then is L, LVIII, XXI, LXXIV, XCI, LXXVIII — all having an odd number of symbols. The second group is XI, XXVI, XXXV, LVII, XLVIII, LXXXVIII — all of which have an even number of symbols.
This rule was a little bit harder than the others, which makes it somewhat more interesting, because some readers came up with alternative rules. As I mentioned, in these problems, as in nature, we are looking for rules that are simple and elegant. More on this issue below.
Problem 6
Follow the rule: QUANTA PUZZLE PHYSICS MOLECULAR BIOLOGY VIDEO
Don’t follow the rule: SCIENCE MAGAZINE INSIGHTS PROBLEMS ARTICLES COMPUTER
All the words in the first group have an even number of letters with curves in them, such as Q, U, P, S, C, O, B, G or D. The words in the second group have an odd number of curved letters.
The first reader to submit all the correct answers was Douglas Felix. It is also interesting and instructive to look at the alternative rules that other readers came up with. (Thank you for the persistence to come up with these ingenious rules, even if they weren’t exactly what we were looking for.)
For problem 5, Anna M. Sheridan came up with something that she confessed was a “lame” pattern: “The first group, when expressed in hex, sorts ascending when sorted in decimal and also in hex (as alphanumeric). The other group doesn’t.” Note that this rule, besides being quite convoluted, also uses the order of the examples within each group, which is expressly forbidden in Bongard problems. In a scientific problem this would be the equivalent of ignoring a natural constraint. Ty Rex’s rule — “There must be a sequence of numbers giving a cyclic pattern of remainders modulo 3 (in this case 2, 1, 0)” — suffers from the same problem.
It is, of course, possible in Bongard problems to come up with a rule that is equally or even more simple and elegant than the one the problem’s designer had in mind. Sheridan’s ingenious rule for problem 6, at first sight, seems equally as good as the correct rule: “Imagine the rain falling from above the text — each of the words in the first sentence has a letter to catch the rain — ‘u’ in Quanta and puzzle, ‘y’ in physics, ‘u’ in molecular, etc. The other line doesn’t have that.” Sorry, Anna, unfortunately the word COMPUTER in the second line has a U that can catch the rain, and therefore rains on your parade. Good try, though! Similarly, scientists often get so enamored of the beauty or ingenuity of their hypotheses that they mourn them when there is evidence to the contrary, a situation pithily expressed by the famous biologist Thomas Henry Huxley: “The great tragedy of science — the slaying of a beautiful hypothesis by an ugly fact.”
I had encouraged readers to contribute their own Bongard-type problems, and Rex and bbctol did so. I was hoping that other readers would attempt these and set up a nice discussion. Please go ahead and still do — I will copy these and other submitted unsolved Bongard-type problems in the comments here for you to solve. I was especially fascinated by the problem submitted by bbctol, which I spent some time with and came up with four to five different candidate approaches, none of which worked. Do you have any hints for us, bbctol? One of the best things about Bongard problems is that every failed approach can become its own original problem using your failed rules.
The Quanta T-shirt for this month goes to Douglas Felix. Thank you to all who contributed. See you again next month for new insights!



