New ‘Superdiffusion’ Proof Probes the Mysterious Math of Turbulence
Introduction
On the afternoon of September 30, 1906, a crowd of 200,000 Parisians congregated near the city’s center to watch the premiere of what would become the world’s most prestigious gas balloon race. Sixteen of the greatest living aeronauts, hailing from seven countries, aimed to travel as far as they could before landing, with nothing but a hydrogen-release valve to control their craft.
As the yellow and amber balloons, each over 50 feet tall, launched into the air, the weather could not have been calmer. But after the sun went down and the spectators dispersed, the winds changed, violently scattering the balloons around Normandy and across the English Channel into England.
The aeronauts were unwittingly participating in an experiment that would alter the course of mathematical physics. Nearly two decades later, a Quaker scientist named Lewis Fry Richardson came across a tabulation of their landing spots in The Aeronautical Journal while studying the effects of turbulent weather. He graphed the balloon data, along with data he had collected himself on the movement of ash after a volcanic eruption and the trajectories of dandelion seeds blown on the wind.
In each case, he observed the same pattern: The turbulent swirling of the Earth’s atmosphere, at both large and small scales, scattered objects with stunning efficiency. Richardson would go on to write a general law about how the process works, one that mathematicians are still struggling to prove more than 100 years later.
Turbulence is one of the greatest mysteries of modern science. The equations that model the flow of fluids — from rivers to air currents — date back two centuries, and they work well when a fluid moves smoothly. But when the flow becomes turbulent, the fluid splits into eddies and whirlpools, which in turn develop smaller swirls. The pattern continues at smaller and smaller scales until molecular collisions eventually prevent eddies from forming. These different-size whorls all influence each other, making it impossible to use the equations to model the fluid’s behavior. We simply can’t know what a given particle in the fluid — or, say, a rubber duck dropped into a raging river — will do next.
By collecting data and simulating fluids on computers, physicists have been able to infer some of turbulence’s qualities. But mathematicians are often unable to prove these statements. The mathematical mystery of turbulence lies at the heart of a $1 million Millennium Prize Problem, one of math’s greatest challenges.

The aim of the Gordon Bennett Cup is to fly a gas ballon as far as possible from the launch site. The 1908 race seen here began in Berlin.
INTERFOTO/Alamy Stock Photo
Richardson formulated another statement about turbulence. He hypothesized that if you drop two rubber ducks in the river, they’ll be carried further and further apart much faster than you might expect. Something in the interaction of all those whorls and swirls will give the ducks a special boost.
Today, this boosted scattering, known as superdiffusion, is seen as a hallmark of turbulence. But until recently, it hadn’t been proved rigorously — even in highly simplified models of fluids.
That finally changed last year. For the first time, three mathematicians have proved that particles dropped in a simplified turbulent fluid do indeed exhibit superdiffusion: They spread out in a predictable but anomalously fast way.
“I think this is going to prove to be one of the most consequential things for the mathematics of turbulence,” said Vlad Vicol, a mathematician at New York University’s Courant Institute who was not involved in the work.
But to Scott Armstrong, a mathematician at the Courant Institute and one of the new paper’s authors, the result is about far more than turbulence. For the past decade, he’s been evangelizing the potential of an arcane mathematical technique. He’s adamant that it’s far more powerful than mathematicians realize — a claim that has been met with skepticism by many of his peers. Now, having used the technique to take on turbulence, he hopes to start changing minds.
Hocus-Pocus
Armstrong didn’t expect his research to involve turbulence. “Ten years ago, I didn’t know what turbulence was,” he said. “I wandered into turbulence after studying a very obscure problem.”
He was analyzing a simplified model of a metallic material using a mathematical procedure called homogenization. In the right scenario, homogenization lets you prove that a system that seems complicated and noisy at small scales actually exhibits simple behavior at a larger scale. It’s essentially a set of arguments to show how small-scale noise averages out over long distances.

For a decade, Scott Armstrong has promoted a mathematical technique he hopes to apply to a broad suite of problems.
Pierre Kitmacher
But homogenization usually only works under very strict assumptions. The small-scale noise has to sit within certain bounds — it can’t be too extreme. This limits how useful homogenization can be: Mathematicians only apply it to analyze the simplest representations of physical systems.
Armstrong, however, saw a beauty and potential in homogenization that others didn’t. He thought that if he honed the technique, it could be used in much noisier settings that hewed closer to reality. “I always thought, eventually, this thing should apply to a lot of problems,” he said. “That this was going to be an important idea if I could ever get it to really work.”
But first he needed a test case. He wanted to use homogenization to prove something no one thought it could handle — a problem that mathematical physicists cared about.
That’s where turbulence came in.
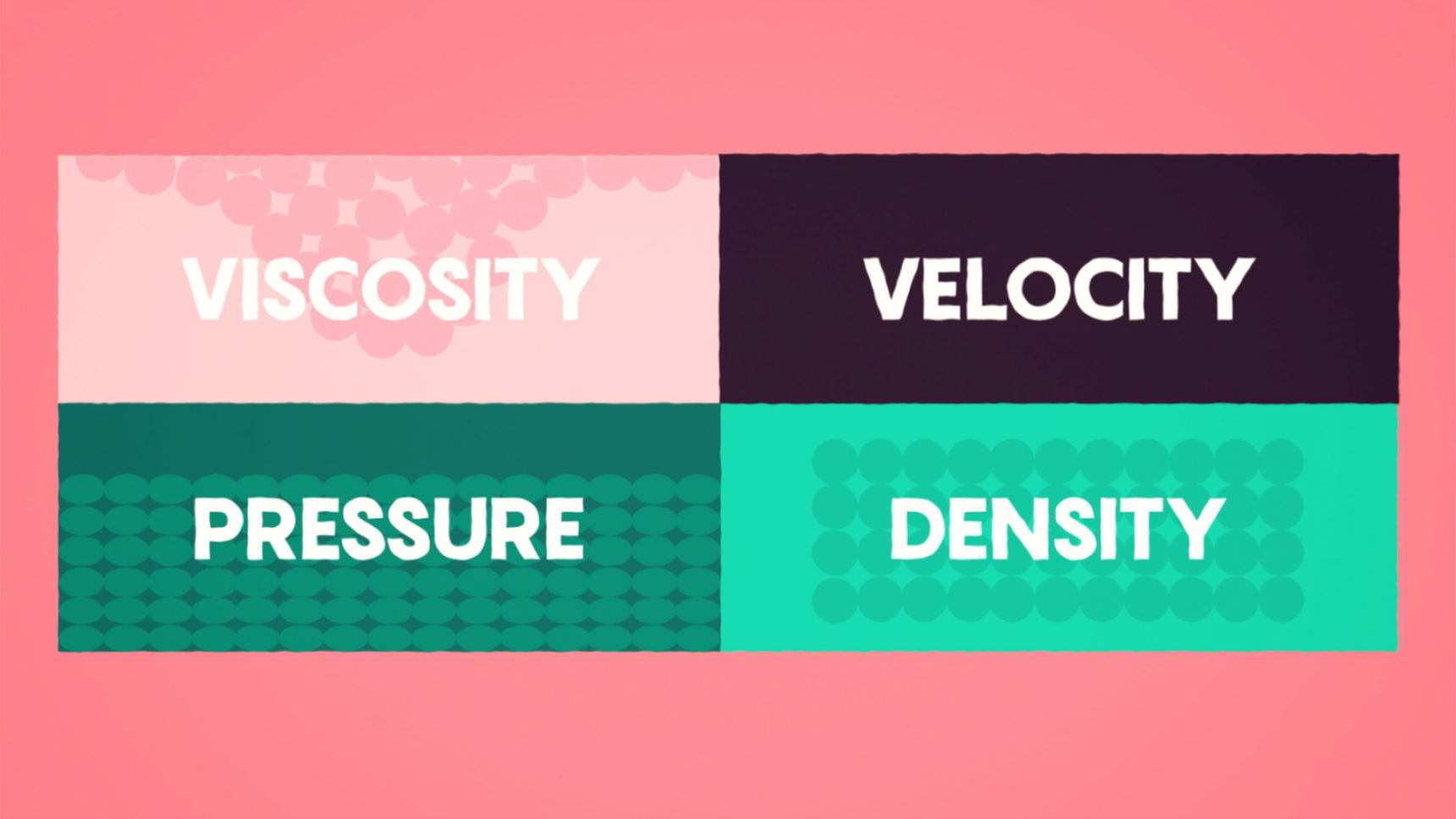
Richardson had hypothesized that in a turbulent fluid, the energy carried by the largest vortices powers slightly smaller ones, and so on down to the smallest scale, where the energy is turned into heat by the friction between the fluid’s molecules. He summarized the idea in a rhyme: “Big whirls have little whirls that feed on their velocity, and little whirls have lesser whirls and so on to viscosity.”
This process, Richardson conjectured, should cause the distance between the two ducks dropped in the river to grow according to a simple equation that’s ubiquitous in classical physics, called diffusion. Only in this case, the cascade of energy from larger to smaller vortices would enhance the rate of that growth — so that in a turbulent fluid, the ducks would exhibit what would later be called superdiffusion.
But as with so many turbulent phenomena, mathematicians weren’t able to prove it.
And so, in the late 1980s, a group of physicists simplified the scenario. They created a stripped-down model of an idealized turbulent fluid. This fluid still exhibited the characteristic eddies and vortices of turbulence, but it was governed by much simpler equations. The team then asked the same question Richardson had: If they dropped solid particles (or ducks) into this imaginary fluid, how quickly would they spread apart?
The researchers conjectured, as Richardson had, that the particles would exhibit superdiffusion, though at a different rate than they would in real fluids. They determined this rate using a technique from physics called renormalization. But renormalization is notoriously lacking in rigor — the renowned physicist Richard Feynman called it “hocus-pocus” — even though it often gives the right answer. Mathematicians have only managed to make renormalization rigorous in a few settings, said Jeremy Quastel of the University of Toronto. “The problem is, it’s a very vague idea,” he said.
And so, while mathematicians were able to prove other statements about how particles spread in the physicists’ idealized fluid, they couldn’t prove their conjecture about superdiffusion. For decades, it seemed that anyone studying turbulence had a choice — either use vague, hand-waving arguments to make strong conjectures as the team of physicists had, or stick to rigorous mathematics and prove things a fraction as significant.
Unless, Armstrong thought, he could get homogenization to put the physicists’ renormalization explanation on more solid mathematical footing.
Step by Step
To show that particles in the turbulent fluid would spread out at a fast enough rate — getting that extra boost of energy from the interactions of the fluid’s vortices — Armstrong first had to gain a better sense of what that spread looked like.
This was where he hoped to bring in homogenization: to show that at larger scales, aspects of the fluid’s behavior could be described with simple equations that would, in turn, tell him the particles’ rate of diffusion. Other mathematicians had their doubts. Researchers had previously attempted to use homogenization to address problems related to turbulence, but they had never gotten very far. So when Armstrong mentioned his goal, he recalled, “they said that I couldn’t prove it.”
But he didn’t give up. He teamed up with his longtime collaborator Tuomo Kuusi, a mathematician at the University of Helsinki — “I’m almost married to him. I mean, how do you describe your best friend?” Armstrong said — along with Ahmed Bou-Rabee, his postdoctoral researcher at Courant. The three mathematicians set out to fortify homogenization so that it would act like a rigorous version of the original renormalization argument.

Tuomo Kuusi recently helped prove that particles dropped in a simplified turbulent fluid exhibit a property called superdiffusion.
Maarit Kytöharju
They started by imagining a very fine grid superimposed on their fluid. They then computed how long particles spent in each square of the grid, on average. In some squares, the fluid acted like a rushing river: Particles tended to sweep straight across the square, spending only a brief period of time there. In other squares, small eddies might push particles around, slowing them down.
The problem was that the numbers the mathematicians calculated might vastly differ from square to square — revealing precisely the kind of small-scale disorder that usually prevented mathematicians from using homogenization.
Armstrong, Bou-Rabee and Kuusi needed to find a way around that.
Ordering Disorder
The mathematicians hoped to show that at slightly larger scales than the one their grid had captured, the fluid’s behavior would be a bit less noisy and disordered. If they could do that, they’d be able to use typical homogenization techniques to understand what was happening at the largest scale.
But other mathematicians thought that even if they succeeded in analyzing those intermediate small scales, the fluid would only look noisier. Before things got smoother, eddies would first merge and interact in even more complicated ways. Trying to show otherwise would be a fool’s errand.

Ahmed Bou-Rabee uses probabilistic methods to study partial differential equations, including those that govern the behavior of fluids.
Courtesy of Ahmed Bou-Rabee
The team decided to try anyway. They started by drawing a slightly coarser grid, in which each square encompassed several squares from the original one. Smaller eddies that had lived in separate squares of the original grid might now get grouped together, changing the average amount of time a particle spent in the new square. Or more complicated behaviors might emerge.
The team once again computed how long particles stayed in each square and how much the numbers associated with adjacent squares might differ. This took painstaking effort: They had to keep track of how the fluid’s behavior in each square would change, and how it would change a particle’s probable motion. They then showed that in this coarser grid, adjacent numbers tended to differ by smaller amounts.
They did this for coarser and coarser grids, until they showed that at a larger — though still relatively small — scale, the fluid looked nice enough for them to use typical homogenization. “You have to do this procedure, which by itself was totally new, infinitely many times,” Vicol said. “The fact that they were able to do this was, from a math perspective, really insane.” It required more than 300 pages of calculation and proof, and took the mathematicians nearly two years.
“It was a very intense experience,” Bou-Rabee said. “There were many Saturday mornings where we’d wake up at 6 a.m. and go to the office to work, and then repeat the next day.”
But once they were able to apply the usual set of homogenization techniques, they had enough information about the fluid at large scales to know that two solid particles dropped into it would spread according to the equation for diffusion. The trio then evaluated the rate of that diffusion and found that it was precisely what the physicists had conjectured decades earlier.
They’d proved the superdiffusion conjecture.
A Long View
The result, which the mathematicians divided into two different papers, provides the first rigorous mathematical understanding of a peculiarity of turbulent fluids: the way they spread particles around with breathtaking efficiency. It’s the first proof of the kind of phenomenon that Richardson observed a century ago in the distribution of balloon enthusiasts across Europe. “You don’t get these sort of definitive results that often,” Quastel said. “I’m pretty impressed — lots of people are pretty impressed.”
Armstrong, for his part, sees the work as a vindication of his ambitions for homogenization. “Nobody expected us to get out of our lane anytime soon,” he said. “So the idea that we would come and then start solving problems in other domains using those methods, there was no sign of that.”
Antti Kupiainen, a mathematician at the University of Helsinki, agreed. “I think even more important is they have a new method, a new way to approach these problems,” he said. In real-life turbulence — which the simplified fluid from the conjecture only modeled in the roughest sense — the scales interact in stronger and more complex ways, leading to more extreme superdiffusive behavior. Perhaps Armstrong, Bou-Rabee and Kuusi’s technique could help researchers chip away at related questions for more realistic models of turbulence, as well as other problems.
Renormalization, after all, is used throughout physics to make sense of systems that exhibit different behaviors at different scales. Armstrong hopes that his techniques can be adapted to prove statements in some of those contexts as well — including particle physics, the area of study where renormalization was first developed.
“I feel like there are so many open possibilities at the moment,” Kuusi said. “I think that this is the last time this will happen to me in my life, and right now I’m going to enjoy the ride.”