The Problem With Dancing Shapes
Last month we discussed the famous Sleeping Beauty puzzle, which is notorious for producing two entrenched camps that both claim to have the right answer and argue endlessly about which is right. So this month we explore a puzzle whose answer, once you find it, is elegant and completely convincing.
Question 1:
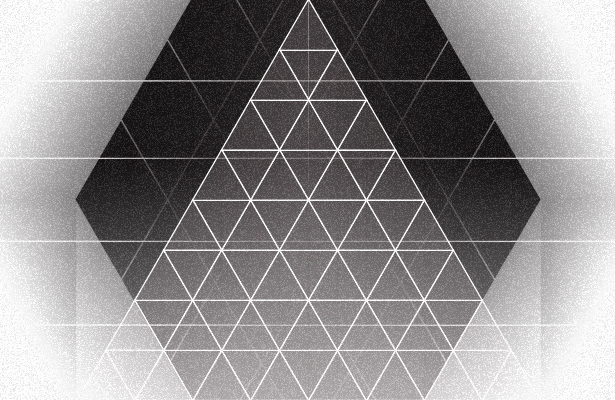
A dance club has set up a social mixer on a “Lucky for Some!” singles night that is designed to overcome shyness and allow people to find someone interesting. On the dance floor they have marked a giant triangle that has been divided into 64 internal triangular cells as shown in the figure. At the start of the dance, 64 ticket holders are each randomly assigned a cell. Then the emcee plays a popular song whose lyrics are 13 lines long. While a line is being sung, participants have to dance in the cell that they find themselves in. At the end of each line, a drumbeat signals that each dancer must move across one of the lines bordering the cell into an adjacent cell. At the end of the song, the lucky dancers whose originally assigned cells are now empty can choose any partner, and the couple is given drinks on the house. (Thirteen, it appears, can be lucky for some!)
What is the minimum number of winners — the dancers for whom the club will have to buy drinks — if the dancers follow the instructions correctly?
Paul Clarke, a high school student from Ireland, wondered if this problem could be generalized to shapes other than triangles, while retaining the property of being guaranteed to be lucky for some. Paul, who now studies at the University of Cambridge, found that some shapes do not work and some are too complicated. However, some do work if the internal lines are drawn correctly (if there are a correct number of internal lines). Below is one shape Paul found that can be made to work.
Question 2:
Imagine the same scenario as in question 1, but now the dance floor is marked with a hexagon, as shown. The internal cells may be hexagons or triangles, but the rules of the dance are exactly the same as before: Dance in your cell until you hear the drumbeat, at which time you cross over a line into an adjacent cell.
Again, what is the minimum number of dancers for whom the club will have to spring for drinks at the end of the song, if the dancers follow the instructions correctly? Can you find a general formula for any hexagon having k internal lines parallel to each side? (The figure shown has four.)
We encourage readers to try other shapes and see if you can come up with interesting results. For example, can you show that a square with k internal lines parallel to each side doesn’t work? You could end up without any winners.
Happy puzzling, and may the insight be with you.
P.S. A few months ago, in the column How to Create Art With Mathematics, we had readers generate beautiful symmetrical patterns based on examples given by author Frank Farris in his book on creating symmetry. Dr. Farris sent me an animated image that he generated by starting with a pentagram with frequencies 2 and -3, and adding in a term with a coefficient of 17 (-17 is one more than a multiple of 6) and an animation parameter. The resulting animation is shown below. I’ve included it here for your entertainment because it does have geometrical figures and dancing, though it is not otherwise related to this month’s problems.
Editor’s note: The reader who submits the most interesting, creative or insightful solution (as judged by the columnist) in the comments section will receive a Quanta Magazine T-shirt. And if you’d like to suggest a favorite puzzle for a future Insights column, submit it as a comment below, clearly marked “NEW PUZZLE SUGGESTION” (it will not appear online, so solutions to the puzzle above should be submitted separately).
Note that we will hold comments for the first day to allow for independent contributions.
Update: The solution has been published here.