Mathematicians Set Numbers in Motion to Unlock Their Secrets

Arithmetic dynamics takes advantage of similarities between objects in number theory, like elliptic curves, and objects in dynamical systems, like the Julia set, to generate new insights about both.
Vignette for Quanta Magazine
Introduction
Joseph Silverman remembers when he began connecting the dots that would ultimately lead to a new branch of mathematics: April 25, 1992, at a conference at Union College in Schenectady, New York.
It happened by accident while he was at a talk by the decorated mathematician John Milnor. Milnor’s subject was a field called complex dynamics, which Silverman knew little about. But as Milnor introduced some basic ideas, Silverman started to see a striking resemblance to the field of number theory where he was an expert.
“If you just change a couple of the words, there’s an analogous sort of problem,” he remembers thinking to himself.
Silverman, a mathematician at Brown University, left the room inspired. He asked Milnor some follow-up questions over breakfast the next day and then set to work pursuing the analogy. His goal was to create a dictionary that would translate between dynamical systems and number theory.
At first glance, the two look like unrelated branches of mathematics. But Silverman recognized that they complement each other in a particular way. While number theory looks for patterns in sequences of numbers, dynamical systems actually produce sequences of numbers — like the sequence that defines a planet’s position in space at regular intervals of time. The two merge when mathematicians look for number-theoretic patterns hidden in those sequences.
In the decades since Silverman attended Milnor’s talk, mathematicians have dramatically expanded the connections between the two branches of math and built the foundations of an entirely new field: arithmetic dynamics.
The field’s reach continues to grow. In a paper published in Annals of Mathematics last year, a trio of mathematicians extended the analogy to one of the most ambitious and unexpected places yet. In doing so, they resolved part of a decades-old problem in number theory that didn’t previously seem to have any clear connection to dynamical systems at all.
The new proof quantifies the number of times that a type of curve can intersect special points in a surrounding space. Number theorists previously wondered if there is a cap on just how many intersections there can be. The authors of the proof used arithmetic dynamics to prove there is an upper limit for a particular collection of curves.
“We wanted to understand the number theory. We didn’t care if there was a dynamical system, but since there was one, we were able to use it as a tool,” said Laura DeMarco, a mathematician at Harvard University and co-author of the paper along with Holly Krieger of the University of Cambridge and Hexi Ye of Zhejiang University.
Moving on a Curve
In May 2010, a group of mathematicians gathered at a small research institute in Barbados where they spent sunny days discussing math just a few dozen feet from the beach. Even the lecture facilities — with no walls and simple wooden benches — left them as close to nature as possible.
“One evening when it was raining you couldn’t even hear people, because of the rain on the metal roof,” said Silverman.
The conference was a pivotal moment in the development of arithmetic dynamics. It brought together experts from number theory, like Silverman, and dynamical systems, like DeMarco and Krieger. Their goal was to expand the types of problems that could be addressed by combining the two perspectives.
Their starting point was one of the central objects in number theory: elliptic curves. Just like circles and lines, elliptic curves are both numbers and shapes. They are pairs of numbers, x and y, that serve as solutions to an algebraic equation like y2 = x3 − 2x. The graph of those solutions creates a geometric shape that looks vaguely like a vertical line extruding a bubble.
Samuel Velasco/Quanta Magazine
Mathematicians have long been interested in quantifying and classifying various properties of these curves. The most prominent result to date is Andrew Wiles’ famed 1994 proof of Fermat’s Last Theorem, a question about which equations have solutions that are whole numbers. The proof relied heavily on the study of elliptic curves. In general, mathematicians focus on elliptic curves because they occupy the sweet spot of inquiry: They’re not easy enough to be trivial and not so hard that they’re impossible to study.
“Elliptic curves are still mysterious enough that they’re generating new math all the time,” said Matt Baker, a mathematician at the Georgia Institute of Technology.
Mathematicians are particularly interested in points on elliptic curves that act like a home base for a special way of moving around on the curves. On an elliptic curve, you can add points to each other using standard addition, but this approach is not very useful: the sum is unlikely to be another point on the curve.
But elliptic curves come packaged with a special internal structure that creates a different type of arithmetic. This structure is called a group, and the result of adding points together using its self-contained arithmetic rules is quite different.
If you add two points on an elliptic curve according to the group structure, the sum is always a third point on the curve. And if you continue this process by, for example, adding a point to itself over and over, the result is an infinite sequence of points that all lie along the elliptic curve.
Different starting points will result in different sequences. The “home base” points are starting points with a very unique property. If you repeatedly add one of these points to itself, it does not generate an infinite sequence of new points. Instead, it creates a loop that comes back to the point you started with.
Samuel Velasco/Quanta Magazine
These special starting values that create loops are called torsion points. They are of immediate interest to number theorists. They also have a striking correspondence to a specific type of point on dynamical systems — and it was this correspondence that really set arithmetic dynamics in motion.
“That’s truly the basis of why this field has become a field,” said Krieger.
Repeating Patterns
Dynamical systems are often used to describe real-world phenomena that move forward in time according to a repeated rule, like the ricocheting of a billiard ball in accordance with Newton’s laws. You begin with a value, plug it into a function, and get an output that becomes your new input.
Some of the most interesting dynamical systems are driven by functions like f(x) = x2 − 1, which are associated with intricate fractal pictures known as Julia sets. If you use complex numbers (numbers with a real part and an imaginary part) and apply the function over and over — feeding each output back into the function as the next input — you generate a sequence of points in the complex plane.
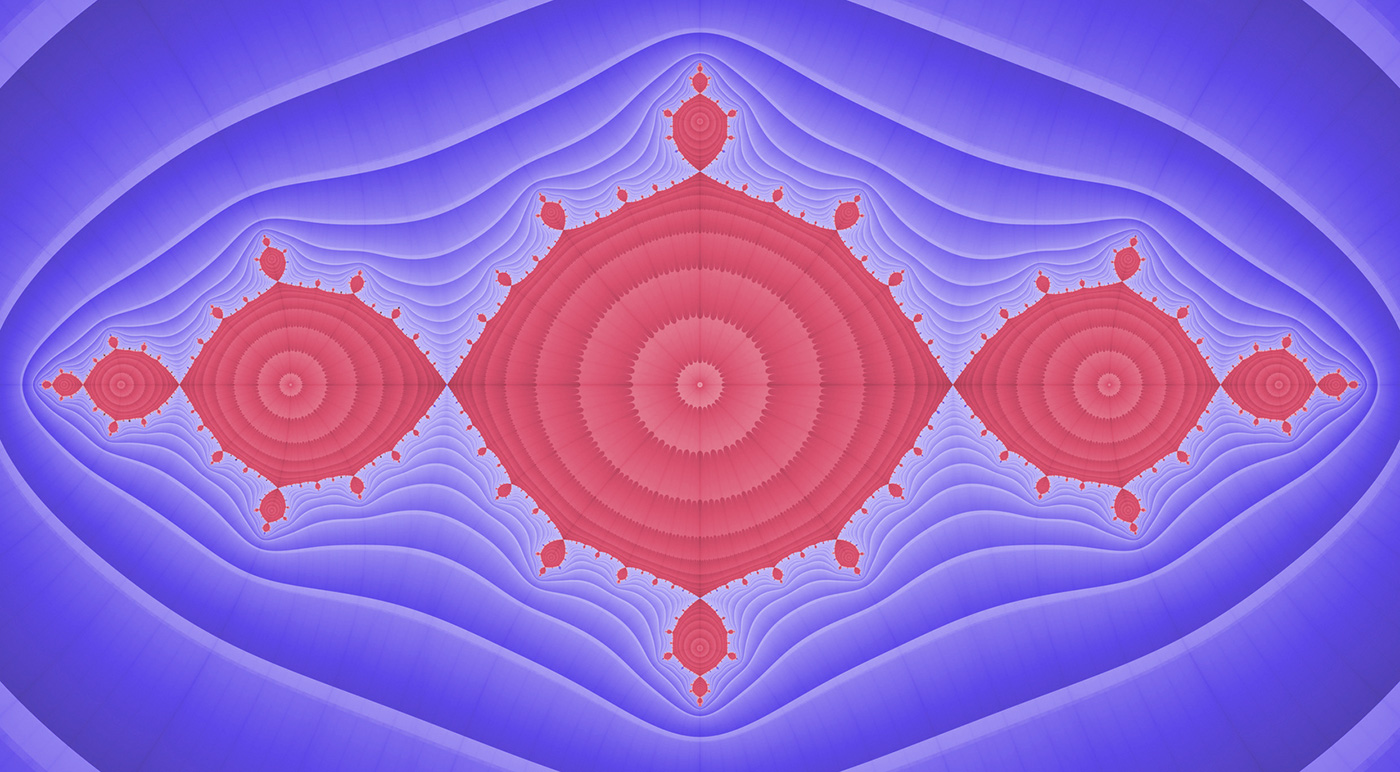
An example of a Julia set.
Quanta Magazine; original: Víctor J. García-Garrido
This is just one example of what’s called a quadratic polynomial, in which the variable is raised to the second power. Quadratic polynomials are the foundation of research in dynamical systems, just as elliptic curves are the focus of a lot of basic inquiry in number theory.
“Quadratic polynomials [in dynamical systems] play a similar role as elliptic curves in number theory,” said Baker. “They’re the ground that we always seem to return to to try to actually prove something.”
Dynamical systems generate sequences of numbers as they evolve. Take for example that quadratic function f(x) = x2 − 1. If you start with the value x = 2, you generate the infinite sequence 2, 3, 8, 63, and so on.
But not all starting values trigger a series that grows larger forever. If you begin with x = 0, that same function generates a very different type of sequence: 0, −1, 0, −1, 0, and so on. Instead of an infinite string of distinct numbers, you end up in a small, closed loop.
In the world of dynamical systems, starting points whose sequences eventually repeat are called finite orbit points. They are a direct analog of torsion points on elliptic curves. In both cases, you start with a value, apply the rules of the system or curve, and end up in a cycle. This is the analogy that the three mathematicians exploit in their new proof.
“This simple observation — that torsion points on the elliptic curve are the same as finite orbit points for a certain dynamical system — is what we use in our paper over and over and over again,” said DeMarco.
Setting a Ceiling
Both Krieger and Ye received their doctorates from the University of Illinois, Chicago in 2013 under DeMarco’s supervision. The trio reconvened in August 2017 at the American Institute of Mathematics in San Jose, California, which hosts intensive, short-term research programs.
“We stayed in a room for five days. We needed to work through some questions,” said Ye.


Holly Krieger (top) and Hexi Ye extended the reach of arithmetic dynamics in new work that counts special kinds of points.
Holly Krieger (left) and Hexi Ye (right) extended the reach of arithmetic dynamics in new work that counts special kinds of points.
Archives of the Mathematisches Forschungsinstitut Oberwolfach; Courtesy of Hexi Ye
During this period, they began to envision a way to extend the crucial analogy between torsion points of elliptic curves and finite orbit points of dynamical systems. They knew that they could transform a seemingly unrelated problem into one where the analogy was directly applicable. That problem arises out of something called the Manin-Mumford conjecture.
The Manin-Mumford conjecture is about curves that are more complicated than elliptic curves, such as y2 = x6 + x4 + x2 − 1. Each of these curves comes with an associated larger geometric object called a Jacobian, which mimics certain properties of the curve and is often easier for mathematicians to study than the curve itself. A curve sits inside its Jacobian the way a piece sits inside a jigsaw puzzle.
Unlike elliptic curves, these more complicated curves don’t have a group structure that enables adding points on a curve to get other points on the curve. But the associated Jacobians do. The Jacobians also have torsion points, just like elliptic curves, which circle back on themselves under repeated internal addition.
The Manin-Mumford conjecture has to do with how many times one of these complicated curves, nestled inside its Jacobian, intersects the torsion points of the Jacobian. It predicts that these intersections only occur finitely many times. The conjecture reflects the interrelationship between the algebraic nature of a curve (in the way that torsion points are special solutions to the equations defining the curve) and its life as a geometric object (reflecting how the curve is embedded inside its Jacobian, like one shape inside another). Torsion points are crowded in every region of the Jacobian. If you zoom in on any tiny part of it, you will find them. But the Manin-Mumford conjecture predicts that, surprisingly, the nestled curve still manages to miss all but a finite number of them.
In 1983 Michel Raynaud proved the conjecture true. Since then, mathematicians have been trying to upgrade his result. Instead of just knowing that the number of intersections is finite, they’d like to know it’s below some specific value.
“Now that you know that they have only finitely many points in common, then every mathematician you would meet would say, well, how many?” said Krieger.
But the effort to count the intersection points was impeded by the lack of a clear framework in which to think about the complex numbers that define those points. Arithmetic dynamics ended up providing one.
Translating the Problem
In their 2020 paper, DeMarco, Krieger and Ye established that there is an upper bound on the intersection number for a family of curves. A newer paper by another mathematician, Lars Kühne of the University of Copenhagen, presents a proof establishing an upper bound for all curves. That paper was posted in late January and has not been fully vetted.
Raynaud’s previous result proved simply that the number of intersections is finite — but it left room for that finite number to be as large as you could possibly want (in the sense that you can always make a larger finite number). The trio’s new proof establishes what’s called a uniform bound, a cap on how big that finite number of intersections can be. DeMarco, Krieger and Ye didn’t identify that cap exactly, but they proved it exists, and they also identified a long series of steps that future work could take to calculate the number.

Laura DeMarco joined with two of her former students to demonstrate how dynamical systems can solve problems about elliptic curves.
Adrian Popa
Their proof relies on a unique property of the Jacobians associated to this special family of curves: They can be split apart into two elliptic curves.
The elliptic curves that make up the Jacobians take their solutions from the complex numbers, which gives their graphs a bulkier appearance than the graphs of elliptic curves whose solutions come from the real numbers. Instead of a wiggly line, they look like the surface of a doughnut. The specific family of curves that DeMarco, Krieger and Ye studied has Jacobians that look like two-holed doughnuts. They break apart nicely into two regular doughnuts, each of which is the graph of one of the two constituent elliptic curves.
The new work focuses on the torsion points of those elliptic curves. The three mathematicians knew that the number they were interested in — the number of intersection points between complicated curves and the torsion points of their Jacobians — could be reframed in terms of the number of times that torsion points from one of those elliptic curves overlap torsion points from the other. So, to put a bound on the Manin-Mumford conjecture, all the authors had to do was count the number of intersections between those torsion points.
They knew this could not be accomplished directly. The two elliptic curves and their torsion points could not be immediately compared because they do not necessarily overlap. The torsion points are sprinkled on the surfaces of the elliptic curves, but the two curves might have very different shapes. It’s like comparing points on the surface of a sphere to points on the surface of a cube — the points can have similar relative positions without actually overlapping.
“You can’t really compare the points on those elliptic curves, because they’re in different places; they’re living on different geometric objects,” said Krieger.
Samuel Velasco/Quanta Magazine
But while the torsion points don’t actually necessarily overlap, it’s possible to think of pairs of them as being in the same relative position on each doughnut. And pairs of torsion points that occupy the same relative position on their respective doughnuts can be thought of as intersecting.
In order to determine precisely where these intersections take place, the authors had to lift the torsion points off their respective curves and transpose them over each other — almost the way you’d fit a star chart to the night sky.
Mathematicians knew about these star charts, but they didn’t have a good perspective that allowed them to count the overlapping points. DeMarco, Krieger and Ye managed it using arithmetic dynamics. They translated the two elliptic curves into two different dynamical systems. The two dynamical systems generated points on the same actual space, the complex plane.
“It’s easier to think of one space with two separate dynamical systems, versus two separate spaces with one dynamical system,” said DeMarco.
The finite orbit points of the two dynamical systems corresponded to the torsion points of the underlying elliptic curves. Now, to put a bound on the Manin-Mumford conjecture, the mathematicians just needed to count the number of times these finite orbit points overlapped. They used techniques from dynamical systems to solve the problem.
Counting the Overlap
In order to count the number of overlaps, DeMarco, Krieger and Ye turned to a tool which measures how much the value of an initial point grows as it’s repeatedly added to itself.
The torsion points on elliptic curves have no growth or long-term change, since they circle back to themselves. Mathematicians measure this growth, or lack of it, using a “height function.” It equals zero when applied to the torsion points of elliptic curves. Similarly, it equals zero when applied to the finite orbit points of dynamical systems. Height functions are an essential tool in arithmetic dynamics because they can be used on either side of the divide between the two branches.
The authors studied how often points of zero height coincide for the dynamical systems representing the elliptic curves. They showed that these points are sufficiently scattered around the complex plane so that they are unlikely to coincide — so unlikely, in fact, that they can’t do it more than a specific number of times.
That number is difficult to compute, and it’s probably much larger than the actual number of coinciding points, but the authors proved that this hard ceiling does exist. They then translated the problem back into the language of number theory to determine a maximum number of shared torsion points on two elliptic curves — the key to their original question and a provocative demonstration of the power of arithmetic dynamics.
“They’re able to answer a specific question that already existed just within number theory and that nobody thought had anything to do with dynamical systems,” said Patrick Ingram of York University in Toronto. “That got a lot of attention.”
Shortly after DeMarco, Krieger and Ye first posted their proof of a uniform bound for the Manin-Mumford conjecture, they released a second, related paper. The follow-up work is about a question in dynamical systems, instead of number theory, but it uses similar methods. In that sense, the pair of papers is a quintessential product of the analogy Silverman noticed almost 30 years earlier.
“In some sense, it’s the same argument applied to two different families of examples,” said DeMarco.
The two papers synthesized many of the ideas that mathematicians working in arithmetic dynamics have developed over the last three decades while also adding wholly new techniques. But Silverman sees the papers as suggestive more than conclusive, hinting at an even wider influence for the new discipline.
“The specific theorems are special cases of what the big conjectures should be,” said Silverman. “But even those individual theorems are really, really beautiful.”
Correction: February 23, 2021
This article has been revised to avoid implying that Lars Kühne’s new work uses arithmetic dynamics.