What's up in
Ramsey theory
Latest Articles
Grad Students Find Inevitable Patterns in Big Sets of Numbers
A new proof marks the first progress in decades on a problem about how order emerges from disorder.
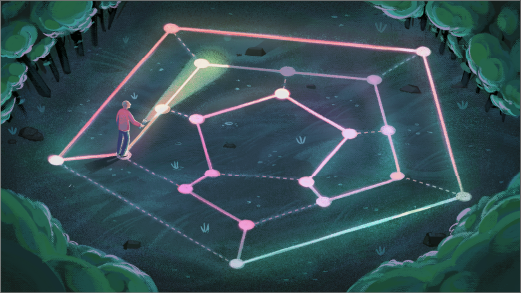
In Highly Connected Networks, There’s Always a Loop
Mathematicians show that graphs of a certain common type must contain a route that visits each point exactly once.
The Year in Math
Landmark results in Ramsey theory and a remarkably simple aperiodic tile capped a year of mathematical delight and discovery.
The Lawlessness of Large Numbers
Mathematicians can often figure out what happens as quantities grow infinitely large. What about when they are just a little big?
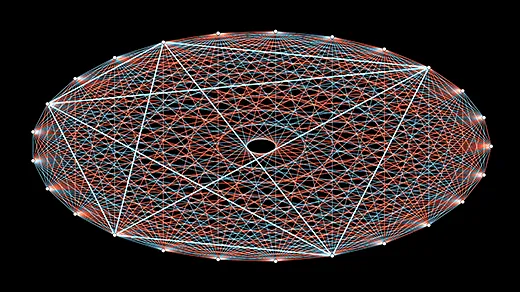
Mathematicians Discover Novel Way to Predict Structure in Graphs
Mathematicians probe the limits of randomness in new work estimating quantities called Ramsey numbers.
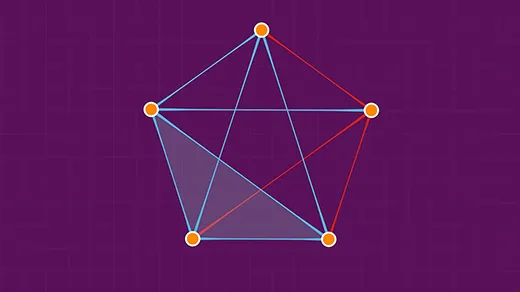
A Very Big Small Leap Forward in Graph Theory
Four mathematicians have found a new upper limit to the “Ramsey number,” a crucial property describing unavoidable structure in graphs.
Why Mathematicians Re-Prove What They Already Know
It’s been known for thousands of years that the primes go on forever, but new proofs give fresh insights into how theorems depend on one another.
Coloring by Numbers Reveals Arithmetic Patterns in Fractions
In a recent paper, two mathematicians showed that a particular pattern is unavoidable when fractions are categorized.
Math’s ‘Oldest Problem Ever’ Gets a New Answer
A new proof significantly strengthens a decades-old result about the ubiquity of ways to represent whole numbers as sums of unit fractions.